Deep analysis of structural characteristics of graphene and its application in electrochemical energy storage

Graphene is widely used in electrochemical energy storage research due to its two-dimensional planar geometric characteristics and unique electronic behavior, improving the output performance of supercapacitors, lithium ion batteries, and increasing the oxygen reduction process (ORR) electrocatalytic activity. A large number of experimental results in the literature have also confirmed the role of graphene in different energy storage applications. At the same time, the researchers also carried out a large number of theoretical calculations to explain the mechanism of action of graphene from the atomic and electronic levels. Xiaobian will lead everyone to understand the theoretical calculation results of graphene in the electrochemical process. The supercapacitor, lithium ion battery and ORR process are typical representatives, learn important conclusions, deepen the understanding of graphene function, and also be new. Graphene-based electrochemical energy storage devices provide research ideas.

From the electrochemical point of view, there are four main functions of graphene in energy storage devices: one is that graphene does not participate in the electrochemical reaction, and the electric charge is stored only by forming an electric double layer with the electrolyte to increase the capacitance. The effect is mainly in the supercapacitor; the other is the electrochemical reaction with the active material, the Faraday current is generated by electron transfer, and the storage place for the electrochemical reaction is provided, such as a lithium ion battery. Or, although no electrochemical reaction occurs, it can be fixed by interaction with the product, and also provides a storage place, such as a lithium-sulfur battery; at the same time, graphene can also provide a catalytic effect for the electrochemical reaction, reducing the electrochemical reaction. The required energy barrier, such as ORR, etc.; and the other is to use the self-conductivity to improve the conductivity of the electrode, reducing the ohmic resistance during charge and discharge. This article focuses on the first three roles.

The electrochemical behavior of graphene in energy storage systems is closely related to its electronic structure. A correct understanding of its electronic structure will be an effective precondition for better use of graphene materials, and it can also provide guidance for the electronic structure adjustment of graphene materials in specific application fields.

Graphene belongs to a triangular Brava lattice consisting of diatomic base points. Since the positions of two adjacent carbon atoms are not identical, the graphene lattice can be divided into two sublattices, each of which is a triangular Brava lattice. The spacing between two adjacent C atoms is 0.142 nm, and the bond angle is 120°, which is the same as the value in the molecular benzene. The C atoms inside the plane are connected to each other by three σ, and the pz orbital of the carbon atoms in the vertical plane forms a delocalized π bond. Figure 1 shows the structure of graphene.

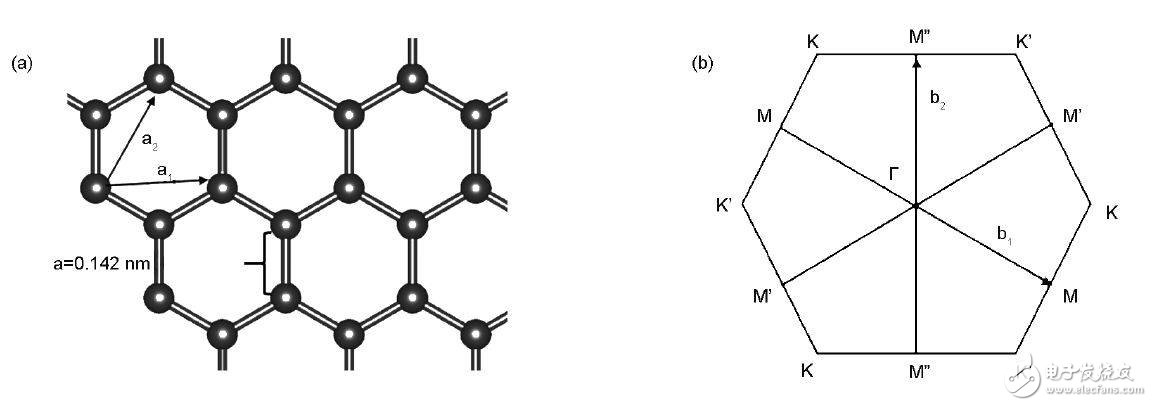
Figure 1 Structural features of graphene: (1) crystal structure; (2) Brillouin area

The three high symmetry points in the Brillouin zone are Τ, K and M, which are the centers, corners, and edges of the hexagon, respectively, as shown in Figure 1 (2).

The energy band structure of graphene is very special, and linear dispersion occurs at K / K' of the reciprocal space, that is, the electron energy of graphene in the vicinity is linearly changed, and the density of the electronic state is zero here. The Fermi level of the complete graphene coincides with the Dirac point, and the π state of the bond near the Fermi level and the π* state of the reverse bond are double degenerate.

In the actual preparation of graphene, it is often difficult to obtain complete graphene, and there are always various defects. At the same time, in order to improve the electronic structure of graphene and give it different chemical properties, the researchers will also adjust the graphene lattice to introduce partial defects or heteroatoms. Common defects on graphene are Stone-Wales (SW) defects, single vacancy (V1) defects, double vacancy (V2) defects, and polyatomic defects, as well as one-dimensional linear defects and grain boundary defects.

Graphene with SW defects does not lose C atoms, only a certain C-C bond is rotated by 90°, and the adjacent four hexagons become two pentagons and two heptagons, so this class Defects are also often referred to as 55-77 defects. The formation of this defect can be very high and the defect location is relatively fixed.

When graphene loses a C atom, a vacancy occurs, creating a V1 defect. Due to the deletion of C atoms, three C atoms with unsaturated dangling bonds appear on the graphene. At this time, the graphene undergoes Jahn-Teller deformation, in which two C atoms are close to each other to form a pentagon, leaving only one dangling bond. . Thus a 5-membered ring and a 9-membered ring appear. The formation of this defect is also very high, but its migration energy barrier is low, and migration can occur on the graphene surface at a lower temperature.

When two adjacent carbon atoms are removed, V2 defects appear on the graphene. At this time, the four 6-membered rings on the graphene become two 5-membered rings and one 8-membered ring, namely 585-V2. The formation of this defect can be comparable to a single vacancy.

In addition to point defects, there are one-dimensional line defects inside the graphene. One is a grain boundary separating graphene crystallites of different orientations, usually composed of a 5-membered ring and an 8-membered ring. Another is the edge of graphene. Due to the dangling keys, the edge structure is usually oriented towards the armchair and zigzga. However, when the two edges have carbon atoms lost, a five-membered ring and a seven-membered ring are produced in the middle of the six-membered ring at the edge.

N atoms are often introduced into the interior of graphene to regulate their electronic structure. N-doped graphene can reduce the formation energy of N-doping by post-processing of graphene or graphene oxide with N-containing precursors, so N-doping is easier in doped graphene than in intact graphene. Deliberate introduction of defects prior to N doping increases the doping effect. For intact graphene, the N atom doping position distribution is relatively dispersed due to the repulsive force between the N atoms, but when there is a defect, the distribution of the region N near the defect will be very concentrated due to the attraction between the defect and N.

B atoms are also often introduced into graphene to adjust their electronic structure. Since B lacks an electron than C, the graphene Fermi level shifts to the lower energy level after B, and does not coincide with the Dirac point. In addition, atoms such as S and P are also used to dope to improve the electronic structure of graphene.

Figure 2 shows the specific structure of the SW defect, V1 and V2 defects. Figure 3 is an electron band diagram of intact graphene, 585-V2 graphene, SW-defect graphene, and graphitized N graphene.

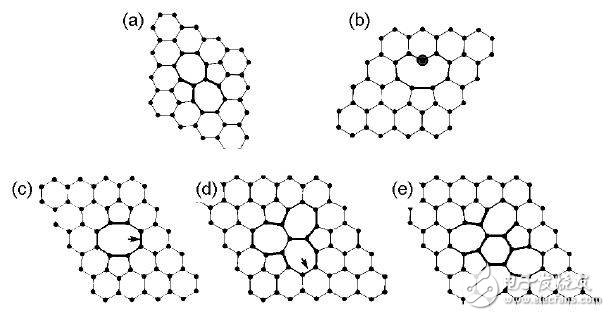
Figure 2 Different types of graphene defects: (a) SW defects; (b) V1 defects; (c) 585-V2 defects; (d) 555777-V2 defects; (e) 555-6-777-V2 defects

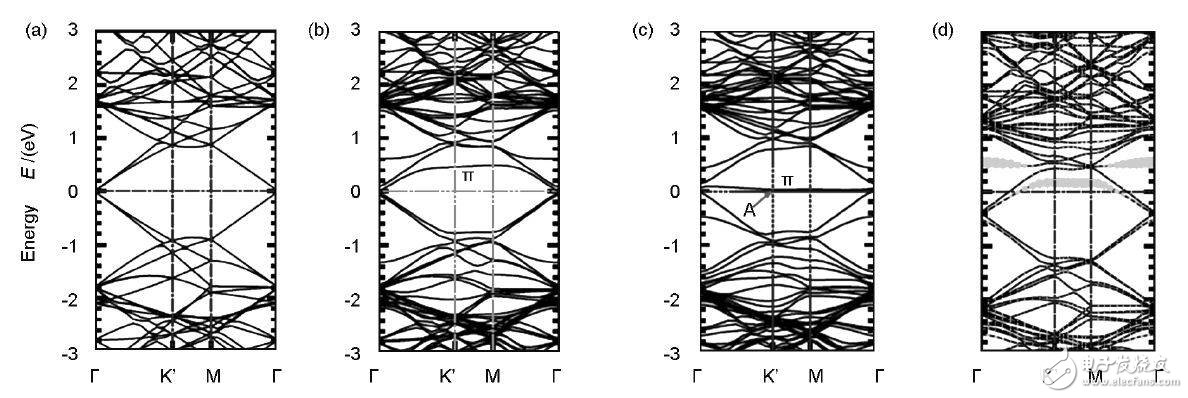
Figure 3 Different types of graphene band structure: (a) intact graphene; (b) 585-V2 defect; (c) SW defect; (d) N graphene

Based on the different roles of graphene in energy storage devices, the current theoretical research progress is discussed from supercapacitors, lithium ion batteries and ORR processes.

Super capacitor

The complete graphene quantum capacitance is very small, and it is difficult to provide sufficient energy for the electrons to store charge during charging or discharging. In order to increase the quantum capacitance of graphene, it is first considered to introduce a defect structure in graphene to improve the DOS of graphene at the Fermi level. For the SW defect, due to the energy band composed of the pz state on the five-membered ring and the eight-membered ring carbon atom at the Fermi level, the band will accommodate additional electrons, thereby increasing the amount of graphene that can be accumulated. At the same time, due to the presence of the pz quasi-local state, the C atoms near the defect in the V2 defect also accommodate additional electrons. The SW defect is very effective in storing charge when it is a negative electrode, while the V2 defect exhibits a good charge storage behavior when it is used as a positive electrode. The substitution of N and B in the lattice will affect the quantum capacitance. The former increases the quantum capacitance in the positive bias range, while the latter increases the quantum capacitance in the negative bias range.

At the same time, the scientists also discussed in detail the effects of the number of layers of graphene, stress and surface folds on the quantum capacitance. Since the density of the single-layer graphene ribbon is low, it is difficult to effectively shield the extra charge generated in the electrode, so the voltage is easily increased rapidly with the increase of the charge, and the capacitance effect is poor. When the number of graphene layers is increased, the number of charges that can be accommodated increases, and the quantum capacitance increases accordingly.

4 is a comparison of intact graphene, SW graphene, V1 graphene, V2 graphene, N-doped graphene, and B-doped graphene quantum capacitors. When graphene has a limited dimension in a certain direction of the plane, a nanobelt is formed. At this time, the electronic structure characteristics and the quantum capacitance have a large change compared with the intact graphene.

The transition metal atom also affects the electronic structure of graphene and produces a strong adsorption effect on the vacancies of graphene.

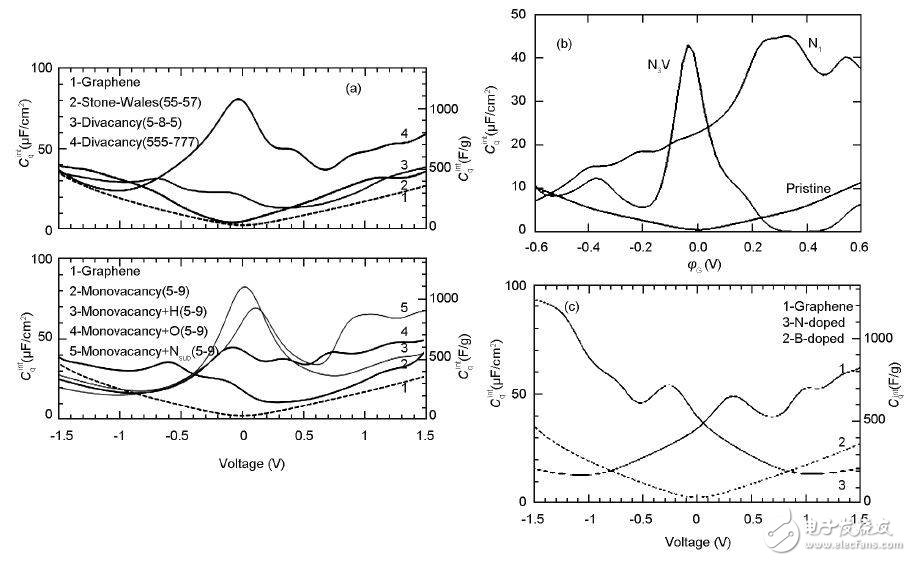
Figure 4. Graphene quantum capacitors of different structures: (a) defective graphene; (b) N-doped graphene; (c) N, B-doped graphene

The theoretical study of graphene in lithium-ion batteries is mainly concentrated in two aspects. The first is the capacity problem, which involves the interaction of Li with graphene, and then the diffusion of Li ions on graphene, which is related to the rate performance of lithium-ion batteries. Tightly connected, mainly including the diffusion of Li in the parallel direction of the graphene surface and the diffusion through the surface of the graphene.

(1) Complete graphene

The interaction of Li on the surface of intact graphene shows that Li is stably present in the middle of the C six-membered ring of graphene. The six carbon atoms forming the ring interact with Li, and the bridging position of Li at two carbon atoms The interaction at the top position of a single carbon atom is very weak. The interaction between Li and C six-membered ring has ionic bond characteristics, and the charge is transferred from Li to graphene. The migrated electrons are mainly concentrated in the region between the Li and C six-membered rings, so the upper region of the Li atom and the CC bond on the graphene. The electrons on the reduction are reduced. When Li is adsorbed on the surface of graphene, the electronic structure of graphene has changed. Since the electrons of Li enter the π* band of graphene, the Fermi level of the system shifts up significantly and enters the conduction band, while the 2s state of Li is located above the Fermi level due to charge transfer. At this time, graphene still has Dirac. Point, but due to the transfer of charge from Li to graphene, the Fermi level becomes slightly higher than the Dirac point.

The scientists found that the adsorption energy of Li and graphene is greater than 0, indicating that Li and intact graphene do not automatically adsorb, and it is difficult to form stable compounds. For a small layer of graphene, Li will automatically react with it. When Li enters the interlayer of a small layer of graphene, the interaction of Li with carbon atoms is affected by the interlayer van der Waals force, and the effect varies with the change of Li content. The calculation results show that the storage capacity of Li in a small layer of graphene is lower than that in bulk graphite. Li can enter any layer of graphene, but can only enter the graphene layer and cannot exist on the surface of the graphene. For two layers of graphene, Li has a very strong intercalation effect when the content is low. As the number of graphene layers continues to increase, Li first enters between the outermost layers of graphene, rather than between the intermediate layers. Li then has different embedding behavior depending on the number of graphene layers, or enters between the same outermost layer, or enters between the other outermost layer or enters the inner layer.

ORR process

Fuel cells can directly convert chemical energy into electrical energy, which has the advantages of high conversion efficiency, high power density and no pollution. The O2 reduction reaction is an important factor that restricts the development of fuel cells. The reaction can take place through two paths, the first being a four-electron process, the O2 obtaining four electrons directly reduced to H2O, and the second being a less efficient two-electron process, with O2 being converted to H2O2. Due to the high utilization efficiency, catalyst research in four-electron processes is very important.

The calculation of the catalytic effect of the graphene shows that the adsorption energy of the O2 molecule and the intact graphene sheet is very small, and the distance between the two is also larger, greater than 2. 6 ? . Although the O2 molecule forms weak ionic bonds with graphene, the complete graphene has no O2 catalytic activity because the energy required for the subsequent steps in the reaction is very high and the generated OOH is also difficult to adsorb on the intact graphene. Since the electronegativity of N is stronger than C, the C atom around N has a positive charge and has a certain spin charge. Studies have shown that when the spin charge density or atomic charge density of a carbon atom is higher than 0.15, it will have the electrochemical activity of ORR. In addition, scientists used the periodic structure graphene model to explore the ORR process of intact graphene and N-doped graphene in detail, as shown in Figure 8. At the same time, the effect of N content on N graphene on ORR process was also calculated. It was found that 4% ~ 5% The N content is suitable for the application.

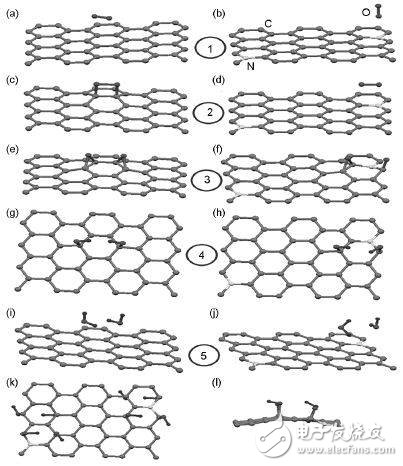
Figure 8 Reaction process of ORR on (left) intact graphene and (right) N graphene

Scientists used a graphene model of graphene to study the catalytic mechanism of N-containing graphene in O2 in an acidic environment. Calculations indicate that OOH is adsorbed on the C atom near the pyridine structure N, one of which interacts with the C atom, and the C atom protrudes from the graphene plane. For pyrrole N, OOH is also adsorbed on the C atom near N. When H is further added to OOH, unstable HOOH is formed on both pyridine N and pyrrole N graphene. The O-O bond length increases and easily becomes two OH, so the ORR on N graphene is a four-electron process. . At the same time, the authors suggest that spin density and charge density are the key factors determining the catalytic effect. Although some C charge density is very high, OOH is more easily adsorbed on C atoms having a high spin density. Compared to the charge density, the spin density is more likely to determine the catalytic activity level, and the charge density only works when the spin density is very small. Figure 9 shows the calculated charge density and spin density distribution for N-doped graphene.

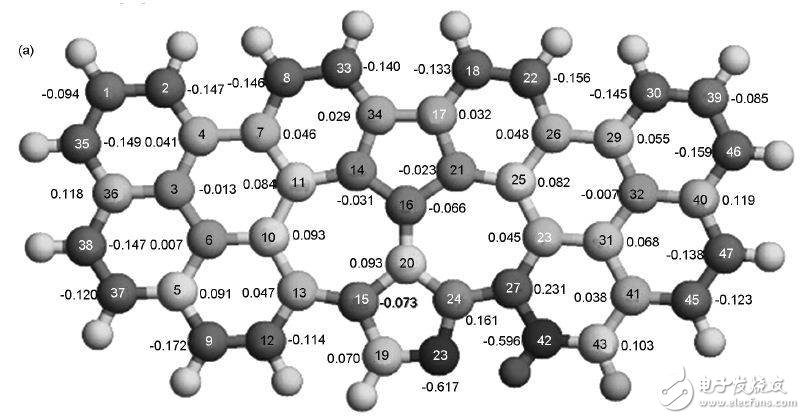
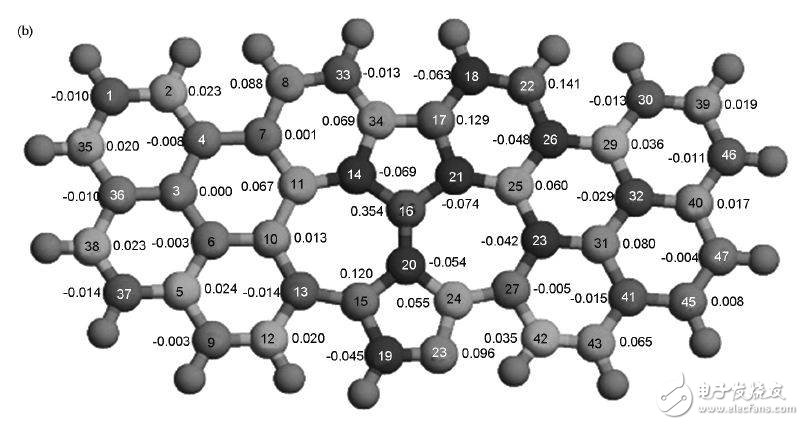
Figure 9 (a) atomic charge density and (b) spin density of N-doped graphene

The negative electrode of the Li-air battery is Li, and the air electrode of the positive electrode is usually porous carbon, which has a very high energy density. The external O2 enters the air electrode and is adsorbed on the surface of the porous carbon, and then O2 decomposes and reacts with the Li ion obtained by the oxidation of the negative electrode. The scientists used the periodic structure model to calculate the catalytic decomposition process of the Li-air battery anode O2 on the surface of N graphene. When the O2 molecule is close to the graphene, an O atom is located above the nearest C atom of N and is inclined toward the graphene plane, and another O is located at the center of the C5N ring, O2 is no longer parallel, and the O-O bond becomes weak.

In addition to the catalysis in the ORR reaction, doping graphene in the O2 precipitation reaction also has significant electrochemical activity. Theoretical calculations show that B-doped graphene can adsorb Li2O2 lower than intact graphene due to electron deficient, and then activate Li-O bond and O2-oxidize to O2 under lower energy barrier. Since the B-doped graphene can greatly reduce the energy barrier of the speed-determining step, the current density of the lithium-air battery can be effectively improved.

In addition, when the state of Li in the bulk Li metal was used as a reference state, the adsorption behavior of Li on graphene was investigated. When this reference state is used, the adsorption energy of Li on the graphene surface is negative, indicating that adsorption can occur, but the capacity is still lower than that of graphite. Coulomb repulsive force exists between different Li atoms on graphene. When the concentration of Li is very low, Li tends to disperse on the surface of graphene, so when the concentration is very low, Li can be adsorbed on graphene. However, as Li increases, Li atoms are separated from graphene by clustering with each other, so the capacity of graphene is lower than that of graphite. The authors analyzed the adsorption of Li clusters on graphene and found that the cohesive energy of clusters adsorbed onto graphene is higher than that of isolated clusters, indicating that the former is more stable. The further development of the agglomeration will form dendrites.

(2) Defects and hybrid graphene

The interaction of Li with the graphene with defects is quite different from that of intact graphene. When Li interacts with the V2-deficient graphene of 585, due to the transfer of Li electrons, the energy level of the near-horizontal band near the Fermi level on the V2 graphene is partially reduced by the electrons. However, the Fermi level is still below the Dirac point. When Li interacts with SW-deficient graphene, the Fermi level rises above the Dirac point and enters the conduction band, while the nearly horizontal band of the SW defect is partially occupied.

Figure 5: Different structures graphene interacts with Li: (a) a small layer of graphene; (b) density density of Li and N-doped graphene

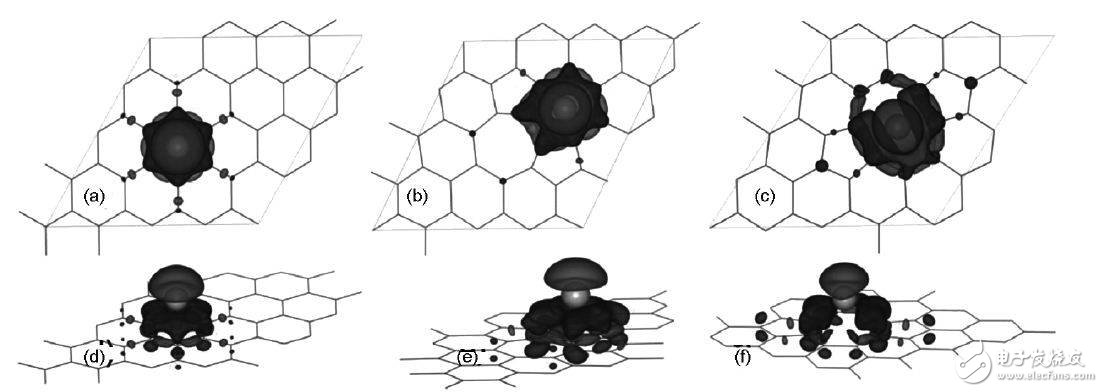
Fig. 6 Electronic differential density map of Li on defective graphene: (a, d) intact graphene; (b, e) V1 defect; (c, f) V2 defect

In lithium-ion battery systems, in addition to the ability to store Li in graphene, an important factor to consider is the effect of graphene on the diffusion process of Li ions, which directly affects the fast charge and discharge behavior of lithium-ion batteries. The diffusion process involves two aspects. Li diffuses on the graphene surface in a direction parallel to the surface, and the other diffuses through the graphene plane in a direction perpendicular to the plane.

The calculation shows that the diffusion barrier of Li on the surface of intact graphene is 0.32 eV, which indicates that Li easily diffuses along the surface of graphene, and graphene as a negative electrode often has good rate performance. The results of the scientists show that when Li diffuses along the top path of the center of the six-membered ring at the top of the six-membered ring—the C—C bond—on the intact graphene, the highest energy hinders the position of Li across the C—C bond. The energy barrier is 0. 311 eV; along the top of the six-membered ring—the top of the six-membered ring at the top of the C atom, the apex of the C atom is an energy barrier, 0.337 eV. When Li diffuses between the layers of two layers of graphene, it also has a lower diffusion energy barrier.

The defects of graphene have an important influence on the diffusion behavior of Li in the plane direction of parallel graphene. When Li diffuses in the V2 defect of the 585 structure, Li is easily fixed in the defect region due to the energy trap generated by the defect. The diffusion barrier on the defect is 0.17 eV, and the diffusion barrier of V1 is 0.24 eV, which is less than the complete graphene (0.331 eV), so the energy trap can greatly reduce the diffusion of Li. Potential energy, further improving the diffusion behavior of Li on the surface of graphene.

Grain boundaries often occur inside graphene, especially in graphene prepared by CVD. Grain boundary defects also have an effect on the electronic state near the graphene Fermi level. Scientists have studied this and found that the most stable adsorption position of Li on graphene is at the grain boundary. The above defect structure has better adsorption capacity for Li than intact graphene. The diffusion behavior of Li atoms in the parallel and vertical grain boundaries of graphene shows that the parallel diffusion energy barrier of Li atoms on the grain boundaries is significantly lower than that of vertical grain boundaries, indicating that it is difficult for Li atoms to cross laterally through the grain boundaries. A microcrystalline area.

The diffusion behavior of Li in the vertical direction of the graphene sheet also has an important influence on the rate performance of the lithium ion battery. When Li diffuses through the intact graphene, the energy barrier is very high due to Coulomb repulsive force, which is 9.8 eV, so it is difficult for Li to pass through the entire graphene surface through the center of the six-membered ring. When there is a vacancy defect, the diffusion barrier is lowered. The experiment calculated the energy barrier of Li passing through different graphenes, and the complete graphene was 10. 2eV, the SW defect was 6. 35 eV, the V1 was 8.86 eV, and the V2-585 was 2. . 36 eV. Therefore, starting from the V2 defect, Li can diffuse through the graphene sheet.

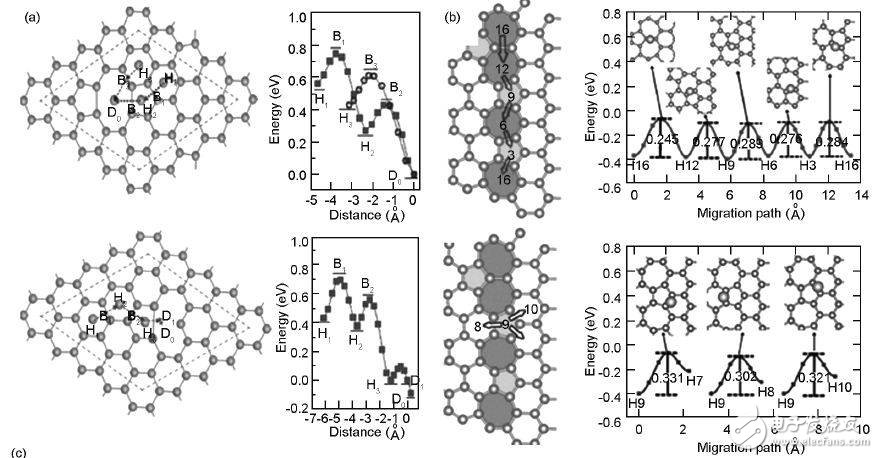
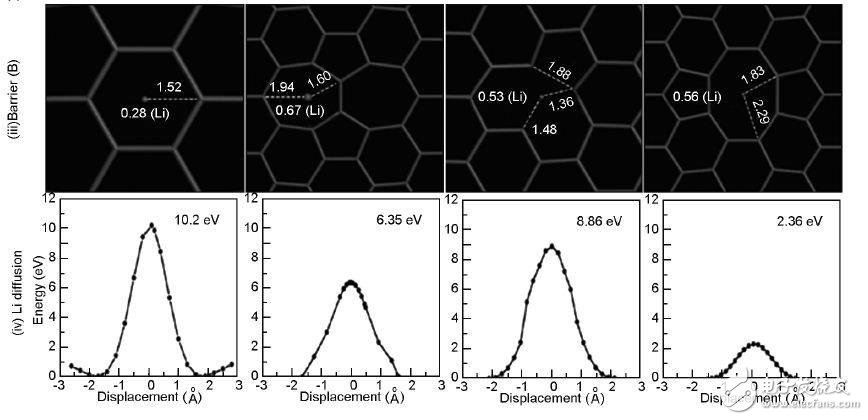
Figure 7 Li ion diffusion process: (a) parallel graphene surface; (b) parallel graphene grain boundary; (c) through graphene plane

E-glass Rod

E-glass rod,Power fittings,Insulator fittings,Polymer insulator

TAIZHOU HUADONG INSULATED MATERIAL CO.,LTD , https://www.thim-insulator.com